Oblique Cone Bottom Tank Volume Calculator - Calculate volume in litres, gallons, cubic feet, bbl, cubic meters

Tank Volume (m³):

Filled Volume (m³):

Tank Volume (U.S. Gallons):

Filled Volume (U.S. Gallons):

Tank Volume (Imp. Gallons):

Filled Volume (Imp. Gallons):

Tank Volume (Liters):

Filled Volume (Liters):

Tank Volume (Cubic Feet):

Filled Volume (Cubic Feet):

Tank Volume (bbl):

Filled Volume (bbl):

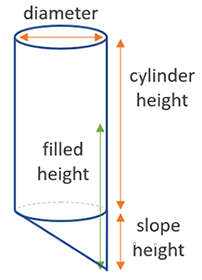
Oblique Cone Bottom Tank Volume Formula

Volume Calculation

The volume of an oblique cone bottom tank is calculated by summing the volumes of the cylindrical section and the conical (slope) section. The formulas are as follows:

\[ V_{\text{slope}} = \frac{1}{3} \pi r^2 H_{\text{slope}} \]

Where:

r = radius (calculated as diameter / 2)
H_slope = height of the slope (conical) section

\[ V_{\text{cylinder}} = \pi r^2 H_{\text{cyl}} \]

Where:

r = radius (calculated as diameter / 2)
H_cyl = height of the cylindrical section

The total volume of the tank, combining the cylindrical and slope sections, is given by:

\[ V_{\text{tank}} = V_{\text{cylinder}} + V_{\text{slope}} \]

Filled Volume Calculation

The filled volume of the tank depends on the height of the liquid relative to the slope height:

If filled height is less than or equal to the slope height:

\[ V_{\text{filled}} = \frac{1}{3} \pi \left(\frac{H_{\text{filled}}}{H_{\text{slope}}}\right)^2 r^2 H_{\text{filled}} \]

If filled height is greater than the slope height:

\[ V_{\text{filled}} = \pi r^2 H_{\text{cyl-filled}} + V_{\text{slope}} \]

Where:

H_filled = filled height
H_slope = height of the slope (conical) section
H_cyl-filled = portion of the fill height in the cylindrical section (H_filled - H_slope)
r = radius (calculated as diameter / 2)